Categories
Discover our Courses
Featured Pathways
More pathways
Banking Essentials - Part I
This pathway will walk us through the basics of banks, starting with some of the different types and their main functions, then starting to look at the regulation faced by the banks, both before and after the Global Financial Crisis.
Greenwashing
Greenwashing is the act of distributing false information about something being more environmentally friendly than it actually is.
More pathways
Ready to get started?
Plans & Membership
Find the right plan for you
Our Platform
Expert led content
+1,000 expert presented, on-demand video modules
Learning analytics
Keep track of learning progress with our comprehensive data
Interactive learning
Engage with our video hotspots and knowledge check-ins
Testing & certification
Gain CPD / CPE credits and professional certification
Managed learning
Build, scale and manage your organisation’s learning
Integrations
Connect Finance Unlocked to your current platform
Featured Content
More featured content
Tackling the Cost of Living Crisis
In this video, Max discusses the cost-of-living crisis currently enveloping the UK. He examines its impact on households as well as the overall economy.
CSR and Sustainability in Financial Services
In the first video of this two-part video series, Elisa introduces us to sustainability. She begins by looking at the difference between sustainability and corporate social responsibility, two terms that can be easily confused.
More featured content
Ready to get started?
Our Solutions
About Us
Testimonials
More testimonials
Mark Homans
Our sky high NPS following learner feedback speaks for itself - this platform has delivered a lot of value.
Fiona Quinn
Learning is accessible and the tool assists in the continued development of all our employees. It is a fantastic tool for our business!
More testimonials
Ready to get started?
Categories
Discover our Courses
Featured Pathways
More pathways
Banking Essentials - Part I
This pathway will walk us through the basics of banks, starting with some of the different types and their main functions, then starting to look at the regulation faced by the banks, both before and after the Global Financial Crisis.
Greenwashing
Greenwashing is the act of distributing false information about something being more environmentally friendly than it actually is.
More pathways
Ready to get started?
Plans & Membership
Find the right plan for you
Our Platform
Expert led content
+1,000 expert presented, on-demand video modules
Learning analytics
Keep track of learning progress with our comprehensive data
Interactive learning
Engage with our video hotspots and knowledge check-ins
Testing & certification
Gain CPD / CPE credits and professional certification
Managed learning
Build, scale and manage your organisation’s learning
Integrations
Connect Finance Unlocked to your current platform
Featured Content
More featured content
Tackling the Cost of Living Crisis
In this video, Max discusses the cost-of-living crisis currently enveloping the UK. He examines its impact on households as well as the overall economy.
CSR and Sustainability in Financial Services
In the first video of this two-part video series, Elisa introduces us to sustainability. She begins by looking at the difference between sustainability and corporate social responsibility, two terms that can be easily confused.
More featured content
Ready to get started?
Our Solutions
About Us
Testimonials
More testimonials
Mark Homans
Our sky high NPS following learner feedback speaks for itself - this platform has delivered a lot of value.
Fiona Quinn
Learning is accessible and the tool assists in the continued development of all our employees. It is a fantastic tool for our business!
More testimonials
Ready to get started?
Introduction to the Monte Carlo Simulation
Abdulla Javeri
30 years: Financial markets trader
Abdulla explains the significance of the Monte Carlo Simulation: what it tries to achieve and how it works. In so doing, Abdulla provides an example using an excel spreadsheet.
Abdulla explains the significance of the Monte Carlo Simulation: what it tries to achieve and how it works. In so doing, Abdulla provides an example using an excel spreadsheet.
Subscribe to watch
Access this and all of the content on our platform by signing up for a 14-day free trial.
Introduction to the Monte Carlo Simulation
4 mins 52 secs
The Monte Carlo Simulation is a technique used to stimulate potential changes to a value, a price, or any number, usually over a number of time periods. It has a wide variety of applications, some of which include: stock prices and inflation rates.
Key learning objectives:
Describe the Monte Carlo Simulation
Outline some of its uses in financial markets
Understand how the simulation works in practice
Subscribe to watch
Access this and all of the content on our platform by signing up for a 14-day free trial.
What is the Monte Carlo Simulation?
- A method used to stimulate a unique path for potential changes to a number
- Based on a random pick from an assumed probability distributed with a mean and standard deviation
- When repeated numerous times it defines the probability distribution of the final outcomes
Under which conditions can the simulation be used?
Essentially it can be used in any area where numbers are subject to change, but where the change is not perfectly predictable, or is subject to some randomness in its movement.What are some of its uses in financial markets?
- Valuation of certain types of options
- Potential changes to the price of assets over time
- In the measurement and management of risk
What does the Monte Carlo method require?
- An average for the expected change
- A standard deviation/volatility
How does the simulation work in practice?
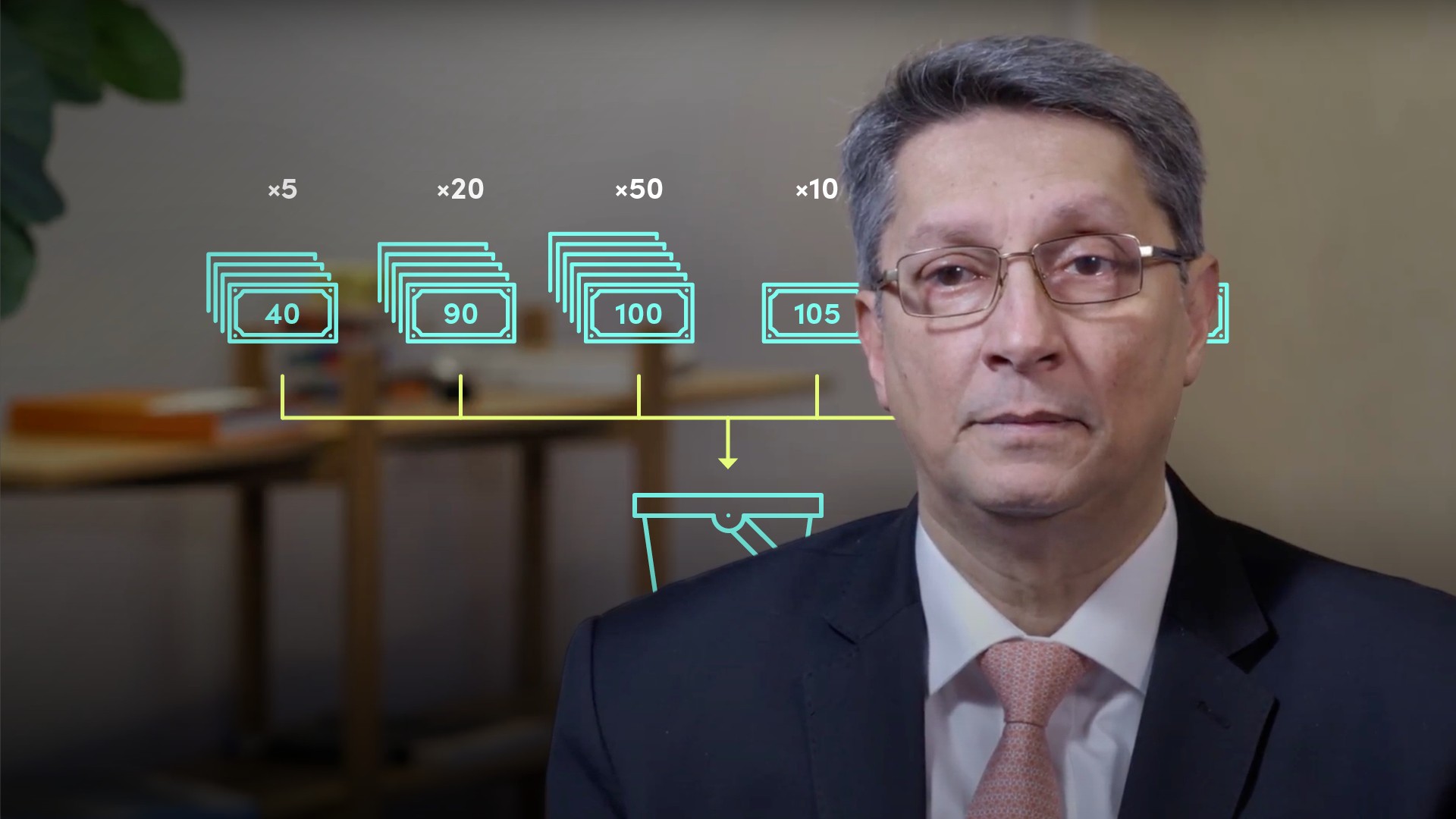
- Today’s value = 100. We want to know what the number could potentially be tomorrow: let's assume that tomorrow we expect the number to remain unchanged, but subject to some variability. Assume that it could be any of the numbers in the figure below - The probabilities associated with those numbers are also shown:
Subscribe to watch
Access this and all of the content on our platform by signing up for a 14-day free trial.
Abdulla Javeri
There are no available videos from "Abdulla Javeri"